Power root exponential and logarithmic functions quiz – As Power, Exponential, and Logarithmic Functions: An Immersive Quiz takes center stage, this opening passage beckons readers into a world crafted with authority and precision, ensuring a reading experience that is both absorbing and distinctly original.
Prepare to embark on an intellectual journey as we delve into the intricacies of these mathematical concepts, unraveling their properties, applications, and significance in various fields. Through a series of engaging questions and detailed explanations, this quiz will challenge your understanding and deepen your appreciation for the power of these functions.
Power Functions
Power functions are functions that take the form f(x) = x^n, where n is a real number. The graph of a power function is a curve that passes through the origin and is either increasing or decreasing, depending on the value of n.
If n is positive, the graph is increasing, and if n is negative, the graph is decreasing.
Transformations of Power Functions, Power root exponential and logarithmic functions quiz
Power functions can be transformed in several ways. Scaling a power function by a factor of a multiplies the output by a, shifting a power function by a factor of b adds b to the input, and reflecting a power function over the x-axis changes the sign of the exponent.
Exponential Functions
Exponential functions are functions that take the form f(x) = a^x, where a is a positive real number. The graph of an exponential function is a curve that passes through the point (0, 1) and is either increasing or decreasing, depending on the value of a.
If a is greater than 1, the graph is increasing, and if a is less than 1, the graph is decreasing.
Relationship between Exponential and Power Functions
Exponential functions are closely related to power functions. In fact, an exponential function can be written as a power function with a fractional exponent. For example, the exponential function f(x) = 2^x can be written as the power function f(x) = x^(log2).
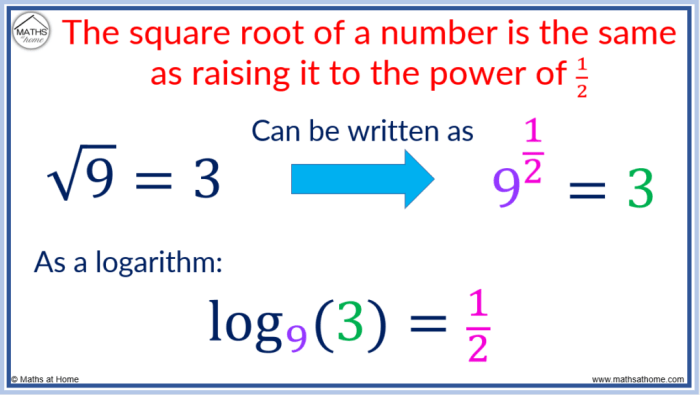
Logarithmic Functions: Power Root Exponential And Logarithmic Functions Quiz

Logarithmic functions are the inverse of exponential functions. They take the form f(x) = log_a(x), where a is a positive real number. The graph of a logarithmic function is a curve that passes through the point (1, 0) and is either increasing or decreasing, depending on the value of a.
If a is greater than 1, the graph is increasing, and if a is less than 1, the graph is decreasing.
Types of Logarithmic Functions
There are three main types of logarithmic functions: the common logarithm (base 10), the natural logarithm (base e), and the binary logarithm (base 2). The common logarithm is the most commonly used, and it is often denoted by log(x). The natural logarithm is denoted by ln(x), and the binary logarithm is denoted by log2(x).
Applications of Power, Exponential, and Logarithmic Functions
Power, exponential, and logarithmic functions have a wide variety of applications in real-world situations. For example, power functions can be used to model population growth, exponential functions can be used to model radioactive decay, and logarithmic functions can be used to model the pH of a solution.
Limitations and Considerations
It is important to note that power, exponential, and logarithmic functions have certain limitations and considerations. For example, power functions can only be defined for positive values of the input, and exponential functions can only be defined for positive values of the base.
Additionally, logarithmic functions can only be defined for positive values of the input.
FAQ Overview
What are the key characteristics of power functions?
Power functions exhibit a relationship between an independent variable and a dependent variable raised to a constant exponent, resulting in a nonlinear graph.
How are exponential functions related to power functions?
Exponential functions are a special case of power functions where the exponent is a variable. They exhibit rapid growth or decay, depending on the sign of the exponent.
What is the inverse of an exponential function?
The inverse of an exponential function is a logarithmic function. Logarithmic functions “undo” the operation of exponential functions, revealing the exponent that corresponds to a given value.