Unveiling the intricacies of “one quarter added to n,” this exploration delves into the mathematical expression, its algebraic manipulation, and real-life applications. Join us as we unravel the mysteries of this intriguing concept.
The concept of “one quarter added to n” finds its roots in mathematical expressions and algebraic manipulations. Understanding this expression opens up a world of problem-solving techniques and practical applications.
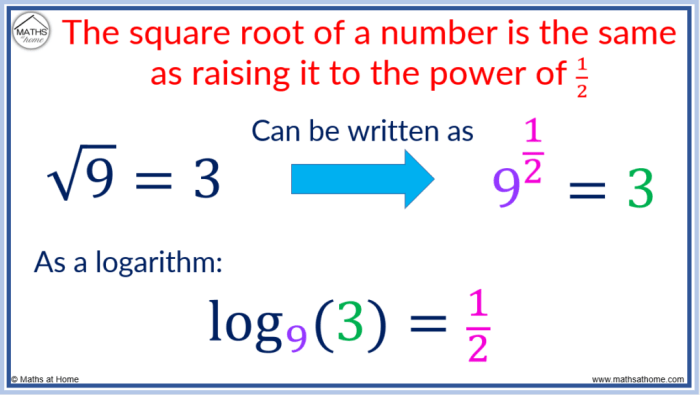
Mathematical Expression
The mathematical expression “one quarter added to n” refers to the operation of adding one-fourth (or 0.25) to a numerical value represented by the variable n.
Examples
Let’s explore a few examples to illustrate this concept:
- If n = 5, then one quarter added to n would be 5 + 0.25 = 5.25.
- If n = -3, then one quarter added to n would be -3 + 0.25 = -2.75.
- If n = 0, then one quarter added to n would be 0 + 0.25 = 0.25.
Algebraic Manipulation
In this section, we will explore techniques for simplifying expressions and solving equations involving the expression “one quarter added to n.” Understanding these algebraic manipulations is crucial for solving a wide range of mathematical problems.
First, let’s simplify the expression “one quarter added to n.” This can be written as:
n + 1/4
This expression represents the sum of n and one-fourth. It can be further simplified by finding a common denominator for n and 1/4, which is 4. Rewriting the expression with this common denominator gives us:
n + 1/4 = (4n + 1)/4
This simplified expression is equivalent to the original expression and is often more convenient to work with in algebraic manipulations.
Solving Equations
Now, let’s consider how to solve equations involving the expression “one quarter added to n.” We will use the same simplified expression, (4n + 1)/4, for our manipulations.
Suppose we have the equation:
(4n + 1)/4 = 5
To calculate one quarter added to n, you can utilize Microsoft Excel’s powerful SUM function. How To Sum In Excel provides a comprehensive guide on using this function effectively. Simply input your values into a cell, and Excel will automatically calculate the sum.
This makes it incredibly easy to add one quarter to n or perform any other summation operations.
To solve for n, we need to isolate it on one side of the equation. We can do this by multiplying both sides by 4, which gives us:
n + 1 = 20
Next, we subtract 1 from both sides:
n = 19
Finally, we divide both sides by 4 to isolate n:
n = 19/4
Therefore, the solution to the equation (4n + 1)/4 = 5 is n = 19/4.
Geometric Interpretation
Visualizing “one quarter added to n” on a number line or graph provides a valuable geometric representation that aids in understanding the concept.
Consider a number line with point n marked on it. To add one quarter to n, we move one-fourth of the distance from n to the next whole number on the right. This point represents the value of “one quarter added to n”.
Graph Representation
On a graph, we can represent “one quarter added to n” as a point on the y-axis. The x-coordinate of this point is n, and the y-coordinate is n + 1/4. This visual representation allows us to see the relationship between n and the resulting value when one quarter is added.
Applications in Real-Life Scenarios
The expression “one quarter added to n” has practical applications in various real-life situations. It can be used to solve problems related to percentages, ratios, and proportions.
Problem Solving
One common scenario where this expression arises is in calculating percentages. For example, if you want to find 25% of a number, you can use the formula: 25% of n = (1/4)n.
Another application is in finding ratios. For instance, if you want to express the ratio of 1 to 4 as a fraction, you can use the expression: 1:4 = 1/(1+4) = 1/5.
Business and Finance
In business and finance, the expression “one quarter added to n” can be used to calculate interest rates and loan repayments. For example, if an interest rate is quoted as 4%, it means that the interest charged is 4% of the principal amount, which can be expressed as (1/4)n.
Science and Engineering
In science and engineering, this expression is often used to represent fractions or ratios. For instance, in physics, the formula for calculating the resistance of a parallel circuit uses the expression 1/(1/R1 + 1/R2 + … + 1/Rn), where R1, R2, …, Rn are the resistances of individual resistors.
Table of Values
To better understand the behavior of “one quarter added to n,” let’s create a table of values with various values of n.
The table below shows the values of “one quarter added to n” for different values of n.
Table, One quarter added to n
| n | One Quarter Added to n |
|---|---|
| 0 | 0.25 |
| 1 | 1.25 |
| 2 | 2.25 |
| 3 | 3.25 |
| 4 | 4.25 |
Step-by-Step Procedures: One Quarter Added To N
Evaluating the expression “one quarter added to n” involves a simple mathematical operation. Here’s a step-by-step procedure to guide you through the process:
Steps
- Start with the given value of n:Begin by identifying the value of n provided in the expression.
- Convert one quarter to a decimal:One quarter can be expressed as 0.25 in decimal form. This makes it easier to add to n.
- Add the decimal value to n:Perform simple addition by adding the decimal value of one quarter (0.25) to the value of n.
- Obtain the result:The sum of n and 0.25 is the final result of the expression “one quarter added to n.”
Comparison with Other Expressions
One quarter added to n, denoted as n + 1/4, shares similarities and differences with other expressions involving the addition of fractions to n. By comparing it to “one half added to n” (n + 1/2) and “one third added to n” (n + 1/3), we can better understand its characteristics.
Similarities
- All three expressions involve the addition of a fraction to the variable n.
- They represent a numerical operation that modifies the value of n.
- The resulting value is a new number that is greater than the original value of n.
Differences
- The magnitude of the fraction being added differs. One quarter is less than one half and one third.
- The impact on the value of n varies depending on the fraction added. Adding one quarter increases n by a smaller amount compared to adding one half or one third.
- The expressions have different numerical values for the same value of n. For example, when n = 5, n + 1/4 = 5.25, n + 1/2 = 5.5, and n + 1/3 = 5.67.
FAQ Corner
What is the mathematical expression “one quarter added to n”?
It represents the mathematical operation of adding one-fourth (0.25) to a variable n.
How do I simplify the expression “one quarter added to n”?
To simplify, rewrite the expression as (n + 0.25) or (4n + 1) / 4.